Datei:Discrete probability distribution illustration.png
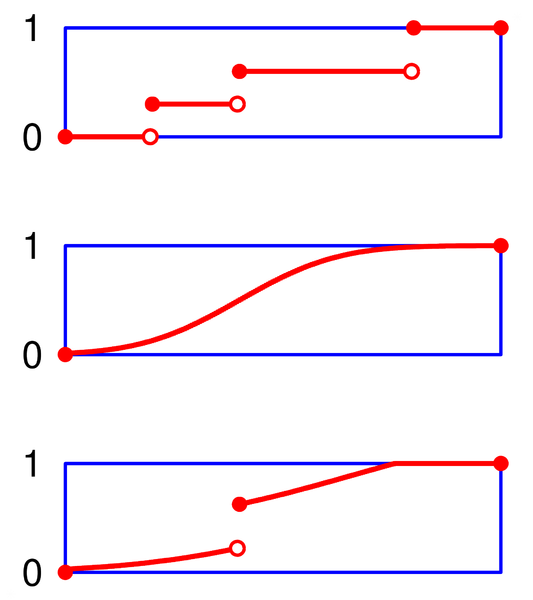
Größe dieser Vorschau: 533 × 600 Pixel. Weitere Auflösungen: 213 × 240 Pixel | 426 × 480 Pixel | 682 × 768 Pixel | 910 × 1.024 Pixel | 1.806 × 2.033 Pixel
Originaldatei (1.806 × 2.033 Pixel, Dateigröße: 44 KB, MIME-Typ: image/png)
Dateiversionen
Klicke auf einen Zeitpunkt, um diese Version zu laden.
| Version vom | Vorschaubild | Maße | Benutzer | Kommentar | |
|---|---|---|---|---|---|
| aktuell | 16:51, 12. Mai 2007 |  | 1.806 × 2.033 (44 KB) | Oleg Alexandrov | {{Information |Description= |Source=self-made |Date= |Author= User:Oleg Alexandrov }} Made with matlab. {{PD-self}} |
Dateiverwendung
Die folgende Seite verwendet diese Datei:
Globale Dateiverwendung
Die nachfolgenden anderen Wikis verwenden diese Datei:
- Verwendung auf be.wiki.x.io
- Verwendung auf el.wiki.x.io
- Verwendung auf en.wiki.x.io
- Verwendung auf es.wiki.x.io
- Verwendung auf fa.wiki.x.io
- Verwendung auf fi.wiki.x.io
- Verwendung auf fr.wiki.x.io
- Verwendung auf he.wiki.x.io
- Verwendung auf he.wikibooks.org
- Verwendung auf hu.wiki.x.io
- Verwendung auf it.wiki.x.io
- Verwendung auf ja.wiki.x.io
- Verwendung auf ko.wiki.x.io
- Verwendung auf pl.wiki.x.io
- Verwendung auf pt.wiki.x.io
- Verwendung auf sr.wiki.x.io
- Verwendung auf su.wiki.x.io
- Verwendung auf sv.wiki.x.io
- Verwendung auf tr.wiki.x.io
- Verwendung auf uk.wiki.x.io
- Verwendung auf vi.wiki.x.io
- Verwendung auf zh.wiki.x.io




